The Bitcoin Dilemma
The Center Cannot Hold: 11
Game theory is the science of multi-agent decision making. It uses mathematics to study the strategic interaction of rational decision makers. Game theory has social, logical, and computer science applications. It also has Bitcoin applications at the personal, social, business, and nation state levels.
Perfect vs Imperfect Information
Sequential games can be organized into two categories, those with perfect information (e.g., checkers) and those with imperfect information (e.g., bridge). A game of perfect information requires that all players know the strategies and payoffs available to the other players, but also know the actions or moves everyone previously made.
For the purposes of this essay we will consider “players” of the Bitcoin game to be those not only holding some Bitcoin but also running a node. We will here define “moves” to be on-chain transactions, or the lack thereof on the public Bitcoin ledger.
If we consider Bitcoin to be an accumulation game, its information is perfect on a surface level, but imperfect much beyond that. It would appear all the node runners know all the strategies and payoffs available to other players, for example they can audit how much Bitcoin they have, and they can even look into how much Bitcoin other addresses have generally on the public ledger, although matching up all the Bitcoin addresses to specific players is an impossible task. Further, all players can see the massive payoff to the common strategy of hodling.
The inverse is also true, players are generally aware that selling sats at any point puts them at great risk of having to buy sats back at a higher or sometimes infeasible price. Most players who sold their hundreds and thousands of Bitcoin during the early years will never possess nearly that number of Bitcoin again. The act of hodling is an emergent strategy that takes players varying amounts of time to adopt, although there was nothing stopping someone from hodling Bitcoin from the beginning. Indeed, Satoshi taught us to hold our Bitcoin from the onset.
The Bitcoin supply is auditable for all the players, the consensus rules are publicly available for every player to verify. All of the moves that have been made, the on-chain transactions are public, although attributing specific moves to specific players is often impossible.
There is also a wealth of more complicated Bitcoin accumulation strategies that have been evidenced on the blockchain, including the wealthiest players triggering sell offs and scooping up cheap Bitcoin at a declined price. More complicated derivatives strategies and a multitude of other Bitcoin accumulation and mining strategies however are not known to all, nor have all of them been developed. This is part of what makes Bitcoin accumulation such a creative and to an extent individualized pursuit. On the whole one could say the Bitcoin does not satisfy our prerequisites for a game of perfect information. Therefore, Bitcoin accumulation is a game of imperfect information.
Complete vs Incomplete Information
Bitcoin could be considered a game of complete information, which requires only that players know the strategies and payoffs available to other players. This is because all rational Bitcoin strategies boil down to increasing the rate of accumulation, and not selling Bitcoin accumulated.
A game of incomplete information becomes a game of imperfect information when one or more players makes a move by nature, or one without stake in a strategic outcome, effectively generating randomness. Proof of stake securities operate like this. Outside of Bitcoin, one can never truly be certain one owns cryptocurrency because it is always susceptible to confiscation or negation via a hard fork initiated by some form of governance. Bitcoin assures you of your property, and does not disenfranchise players through upgrades. Although one could argue there are many moves by nature in Bitcoin as well, in the sense that the markets are irrational, but they don’t appear to be random.
Alpha-Beta Pruning
Chess is a combinatorial game of perfect information. The combinatorial subcategory of games denotes those in which the optimal strategy is based on a myriad of possible moves. Although chess is a game of perfect information, a provable optimal unifying strategy for chess has not been found. A novice chess player may experience information paralysis or a data overload due to the game’s combinatorial nature. If Bitcoin is combinatorial, the myriad of moves are all possible economic tradeoffs one could make to acquire it, and the optimal strategy is to buy and hold Bitcoin and never sell it.
Alpha—beta pruning is a type of computer program that uses a search algorithm. The program stops evaluating a move when it is found to be worse than one that was examined before. Artificial neural networks train through reinforcement learning to make games like chess more computationally tractable. Many people who come to Bitcoin make strategic mistakes at first, those with good internal alpha-beta pruning processes tend to forgo trading stocks and other assets, purchasing cryptocurrencies, and purchasing much of anything at all outside of Bitcoin.
Cooperative
A game is cooperative if players can form alliances that are externally enforced. Accumulating Bitcoin is in a broader sense cooperative. Anecdotally, one can probably stack more Bitcoin working for a Bitcoin company, which presents further opportunities to work with more people who have Bitcoin than working at a grocery store, which presents likely no growth opportunities, at a wage that is inflated away. The Bitcoin network is full of companies and mining firms who are reallocating capital in ways that are mutually beneficial to employers and employees alike. In this way Bitcoin accumulation incentivizes Bitcoin holders working together to bring products of value to market.
Games in which players can form agreements but only through self-enforcement, (e.g., a credible threat) are non-cooperative. Cooperative games can be studied with coalition forming predictions, joint group actions, and collective payoffs. Bitcoin exchanges, miners, mining pools, and businesses are all diligently studied in this way.
The Prisoner’s Dilemma
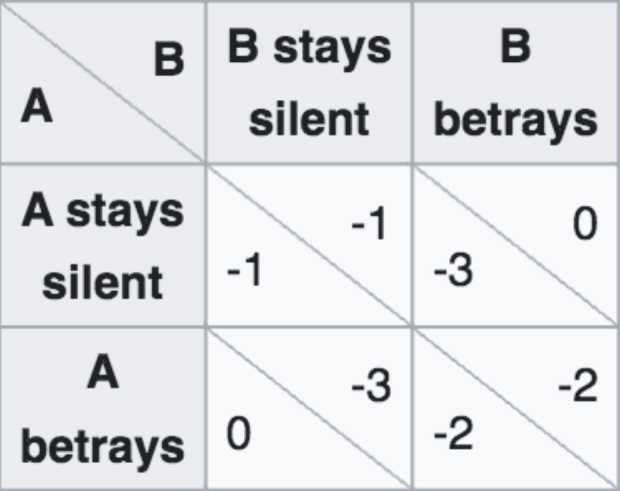
The Prisoner’s Dilemma is a game which proves why two rational individuals acting in their own self interest may not cooperate to achieve an optimal outcome. This theoretical game is played as follows: Two friends are arrested for a crime. They are held in solitary cells without means of communicating with one another. The prosecutor’s do not have enough evidence to imprison both people on the principal charge, but they can both be imprisoned for lesser charges. Each person is offered the same bargain, in their own cell, at the same time. They are each given the option to cooperate with one another by remaining silent, or to betray, and testify that the other person committed the crime.
In this game there are four possible outcomes*:
1) A and B both betray each other, and each of them serves two years in prison.
2) A betrays B, but B remains silent. A is freed, while B serves three years in prison.
3) A remains silent, but B betrays A. A serves three years in prison, while B is freed.
4) A and B both remain silent. Both of them will serve only one year in prison for the lesser charge.
* It is implied that the decision to betray or remain silent will not affect a player’s reputation or well being in the future. Imagine there are no repercussions outside of the possible prison sentences. The strategy to this game changes when players play multiple times in a row, but that is a topic for another essay.
Below is the payoff Matrix for Prisoner’s Dilemma.
Payoff Matrix for The Prisoner’s Dilemma
Description of the Payoff Matrix
We can see from this payoff matrix that to achieve the optimal outcome one player must betray the other. That means that two purely rational players will betray each other. Though there is a slight advantage in both players remaining silent (a shorter prison sentence), the risk of your partner defecting is very high. The Prisoner’s Dilemma is a non-cooperative game, as the players have no information about the other player’s respective choice, so there is no means of agreement, but even if they could agree, there is no external enforcement agent binding players to their agreement. The Prisoner’s Dilemma is a game of complete information in that each knows the payoffs and strategies available to the other, but also one of imperfect information, because, at the decisive moment, one does not know whether their co-player has kept silent or betrayed.
The Bitcoin Dilemma
How does this apply to Bitcoin? A similar model can be found on any scale in our Bitcoin game. If you take any two individuals, businesses, competing nations, large corporations, any entities for whom the goal is to acquire more capital and enrich themselves, they are all witting or unwitting participants in the Bitcoin Dilemma. Players can either choose to accumulate Bitcoin at any moment or defer to a higher price.
Payoff Matrix for The Bitcoin Dilemma
On September 7th, El Salvador became the first country to make Bitcoin legal tender. The world is watching this experiment, and Bitcoiners are eager to see what country will be next to adopt Bitcoin as nations are forced to compete through accumulating or be left behind.
In September, Edward Snowden took to Twitter to urge nations to embrace Bitcoin. After El Salvador made Bitcoin legal tender, the game-theoretic prisoner’s dilemma of nation’s Bitcoin adoption started playing out in global geopolitics. The famous whistleblower highlighted that Bitcoin favors those entities (at any level) that adopt it early, thereby putting pressure on other parties, which will be penalized for being laggards.
Adoption works like this on an individual level too, any insular community ignorant of Bitcoin can live some time without noticing the effects of not owning Bitcoin, although as soon as one member of the community begins to own the hardest money known to us, and that stack begins to appreciate, those who notice their success are presented at every moment with a choice to buy Bitcoin now or put off buying Bitcoin, only to buy it later at a higher price.
31 October 2021
Read The Center Cannot Hold: 10: “The Bitcoin Constant”
Read The Center Cannot Hold: 9: “Schrödinger’s Bitcoin”
Read The Center Cannot Hold: 8: “Bitcoin Is The Singularity”
Read The Center Cannot Hold: 7: “Bitcoin Will Advance Science And Technology”
Read The Language of Bitcoin: 6: “Michael Saylor Interview: The Predator Prey Dynamics of Bitcoin”
Read The Language of Bitcoin: 5: “Bitcoin Has No Competition”
Read The Language of Bitcoin: 4: “Bitcoin And Existential Risk”
Read The Language of Bitcoin: 3: “Bitcoin: The First and Final Rival Money”
Read The Language of Bitcoin: 2: “Bitcoin Alleviates Future Uncertainty”
Read The Language of Bitcoin: 1: “BTC Is The Best Explanation For The Way Money Is”